Matrix Application 2-Computer Graphics

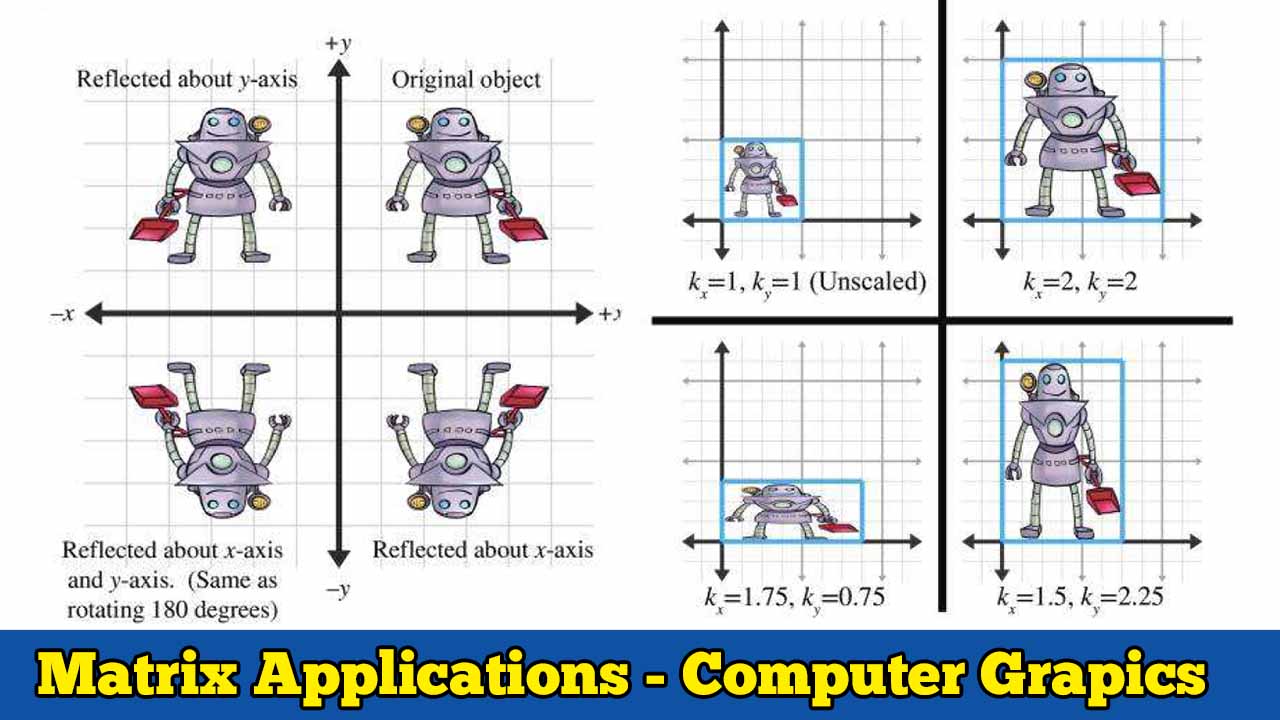
       A Matrices is a two dimensional arrangement of numbers in row and column enclosed by a pair of square brackets or can say matrices are nothing but the rectangular arrangement of numbers,expression,symbols which are arranged in column and rows. Matrices find many applications in scientific field and apply to practical real life problem. Matrices can be solved physical related application and one applied in the study of electrical circuits, quantum mechanics and optics, with the help of matrices, calculation of battery power outputs, resistor conversion of electrical energy into another useful energy, these matrices play a role in calculation, with the help of matrices problem related to Kirchhoff law of voltage and current can be easily solved. Matrices can play a vital role in the projection of three dimensional images into two dimensional screens, creating the realistic decreeing motion. Now day’s matrices are used in the ranking of web pages in the Google search. It can also be used in generalization of analytical motion like experimental & derivatives to their high dimensional. The most important usages of matrices in computer side application are encryption of message codes with the help of encryptions only, internal function are working and even could work with transmission of sensitive & private data. Matrices are also used in geology for seismic survey and it is also used for plotting graphs. Matrices used in Statistics plays a vital role in scientific studies. Matrices are also used in robotics & automation in terms of base elements for the robot movements. The movements of the robots are programmed with the calculation of matrices ‘row &column “Controlling of matrices are done by calculation of matrices.